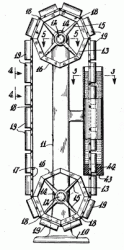
I'm getting tired of checking buoyancy machines which are supposed to be
PMMs. The most recent example was published by a french inventor who sent
me the URL of his two patented machines, one dating from April 2003. Buoyancy
machines are by far the most abundant species of perpetual motion machines.
Unfortunately abundance is no guarantee for workability.
The idea of using buoyancy forces to keep a PMM moving is old and concepts
are abundant. There are two main classes of buoyancy PMMs which can be briefly
described as:
-
partly immersed system, with or without detachable masses.
-
completely immersed system, with or without detached masses, but with changeable
volume of the masses.
Pressure, Gravitation and Buoyancy
It is a strange thing around buoyancy. Only few people are able to describe
how this effect is caused. Buoancy seems to be appealing because of the hidden
secret of its modus operandi. But there are no secrets except the fact, that
obviously most inventors of buoyancy machines missed their physics lesson
or had bad teachers - both possibilities are not a good starting point to
do engineering. Here you find in a nutshell all you need to know about buoyancy.
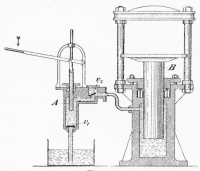 |
|
Let's start with pressure. Pressure is defined as force per
surface area. Usually, textbooks point out that pressure acts uniformly
in liquids. If pressure is applied, it spreads all over the liquid and the
force of pressure acting onto the walls of the container is everywhere the
same. This often is illustrated by the principle of the hydraulic press.
What textbooks don't explicitly write in this context: the applied pressure
distributes uniformly, but the second component, which causes pressure, namely
gravitation, adds a second pressure component. This causes higher pressure
at the bottom of a device and lower pressure at the top. |
How is buoyancy caused? If an object is partly or totally immersed in a liquid,
the pressure applies on that object. Pressure applies from all directions
and always results in a force acting normal to every surface. We can see
that only the vertical components of the pressure forces sum up to a resulting
force which are able to cause an object floating. But first, let's have a
closer look at pressure acting on an object!
If you have read until this point, you should wonder, why up to now, the
mass of the immersed object was not taken into account. Up to now, I've only
described dependencies from the volume of the object. This is a very important
result: The buoancy force is independent from mass or shape, but only from
the liquid's density and the object's volume..
We are familiar with the fact that boats usually float in water, stones sink
and submarines can decide. These different sorts of behavior depend from
the density of the object, not from its absolute mass.
Some conclusions of this section are:
-
Buoyancy is closely connected to gravitational force. In a spacecraft, at
zero gravity, buoyancy effects cannot be observed.
-
The principles of buoyancy apply on every type of liquid and objects. Air
can be regarded as a liquid of very low density in which "lighter" objects,
like ballons, can float. Sometimes the bouyancy force in air must be taken
into account when a machine is to be analyzed.
The Floating Rope
A classical PMM suggestion is given by a light, well-greased floating rope
which is partly immersed in a U-tube. The rope is expected to be in perpetual
motion due to the buoyancy force inside the asymmetric part and the gravitational
force outside. A lot of discussions have been made about this machine.
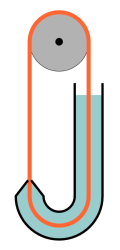 |
|
Ord-Hume gives this explanation (p.108), why the design of that mechanism
is flawed:
"The system breaks down once more on the grounds that the mechanism demands
the relationship of air and water in an unrealistic and impractical situtation.
Aside from the friction in the bearing of the pulleywheel, the well-greased
rope has to be drawn through a watertight constriction which has to exert
some appreciable pressure on the rope in order to retain the liquid in the
tube. If one tries to add more 'power' to the lifting side of the system
by lenghtening the longer side of the J-tube, the weight of water is also
increased, the tendency to leak between rope and constriction is increased
and so the constriction must hug the rope yet tighter, increasing the friction
still further. As if all this was not enough, the friction incurred in bending
and unbending the greased rope in the water would also be appreciable. The
efficiency of this suggested machine would be considerably less than the
value 1.0 - and remember that perpetual motion would only ensue if the efficiency
were to exceed 1.0." |
Sounds plausible, but misses the point. Ord-Hume argues by friction. But
even if there were no friction, the whole device would not work in the intended
way.
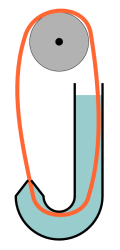
The inventor of this floating rope mechanism rises expectations by a drawing
which does not come very close to reality. Have you ever observed a floating
rope? If so, could the drawing be correct? If the rope were floating, why
doesn't it float at the U-shaped bottom of the tube?
 |
|
 |
|
 |
How a stiff rope
would float |
|
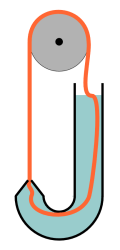
A flexible rope would
float like this... |
|
...except it is
sharply bent. |
 |
|
Still,the reason why this device won't work, is unclear. Here is a very
simple explanation:
-
The U-shaped portion of the tube will generate a symmetric buoyancy force
on both ends of the rope which compensate. Consequently, Mr. William Chaper
omitted this unproductive detail and constructed his machine with the vertical
portion immersed only. In 1870, he published the design.
-
The asymmetric vertical portion of the tube does not generate a buoyancy
force at all, as the pressure acts symmetrically and vertically to the surface
of the rope. The pressure cannot act on the top or bottom portion, as they
are not present and thus cannot be exposed to the pressure difference between
the surface of the liquid and the lower part of the tube.
|
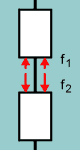
The Floating Chain Machine
For the benefit of the inventor, we reduce the space between the floats and
assume that the difference f2-f1 is very close to zero.
All these forces sum up to a force little more than zero, pointing down.
Let's neglect this force. Will the machine work under these conditions?
Up to now, we have not considered the lowest and the topmost immersed float.
The lowest float is not supported by buoyancy from the bottom. Thus this
float is pushed downwards by pressure and gravity. We assumed that the sum
of forces between the gaps of the floats should be neglected. But we cannot
neglect the difference of forces between the top of the bottom float and
the bottom of the topmost float. Again the principle of the machine fails.
Really?
Have you read the "Methods" chapter? Remember the first hint: Don't
be gullible, be sceptic! Do you believe all what you read, if it is explained
good enough? If my explanation about the gaps were correct, why does the
machine not operate in the opposite direction?
An Advanced Buoyancy Problem
We have seen how perpetual motion concepts of the simple float-on-chain type
can be analyzed. Sometimes, a buoyancy PMM is invented, where we can assume
that the inventor has made similar considerations. Here, I'd like to discuss
a machine principle, that was suggested by Ralph W. Gilmore in 1974. He got
the US patent no. 3,857,242 for it. The principle is based on an endless
chain with floats, but the decisive detail is the fact that the float can
be detached from the chain.
We have learned how this machine shall work. I'd like to point out that the
inventor has cleverly avoided to move the float vertically at the botton
into the tank. Instead, he pushes it horizontally through a valve mechanism.
So only the pressure force acting on the front has to be overcome. We assume
-
the vertical component does not cause any frictional losses by bending the
object or pressing it against the rim of the valve mechanism.
-
The valve mechanism does not leak and by sophisticated construction opens
and closes without the need of power.
Will this machine work? And if not, can you give exactly the reason why?
The Sophisticated Buoyancy Machine
I'd like to point out that I'm not willing to use formulae here, except a
few additions and subtractions. The flaw of a principle must be made obvious
without mathematics.
We focus on the second class of buoyancy machines, as they are more difficult
to understand. For the convenience of the reader and the benefit of the inventor,
we ignore frictional losses. A first drawing illustrates the machines' principle:
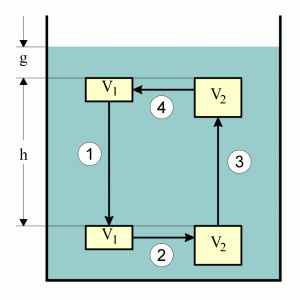 |
|
Attached to a chain, several bodies with changeable volume are expexted
to move in, say counterclockwise direction. The volumes are:
-
V1 body sinks down
-
V2 body raises
The whole mechanism is completely immersed in a suitable liquid, e.g. water.
The mass of the bodies and the volumes V1 and V2 are
chosen in a way, that the abovementioned property holds. It is easy to understand
that the idea can work with any desired number of bodies, so it is sufficient
to analyze the way of one. A sort of circular process is expected to happen:
-
body has volume V1, therefore sinks down
-
body's volume is increased by an inner mechanism. New and larger volume is
V2
-
body raises
-
body's volume is decreased by an inner mechanism to volume V1
|
We assume that the mechanism inside the body works without losses of energy
(e.g. by friction) so that the two changes of volume add up to an energy
of zero. The conclusion is, as both steps 1 and 3 are caused by buoyancy
effects, the process should generate an excess of mechanical energy that
can be drawn for useful work.
This idea is very appealing and more than one patent has been issued for
machines of this kind.
Some readers might stop here and start building a perpetual motion machine
on this principle. I kindly ask them to read until the end of this page,
as they have a good opportunity to avoid frustration. In order to keep the
analysis as simple as possible, we look at several aspects of this mechanism
separately. By this, it should be easy to identify the "magic" portion of
the device or the process resp., which causes the generation of energy.
Circular Path of the Mass
First, we should have a critical look at the circular path of the mass. All
patents and suggestions of this class of machines which I know up to now,
assume that the path of the masses is closed and the shape, size or other
mechanical conditions of the mass are the same in the same location on its
path. As the liquid shall not be considered at the moment, we can easily
see that the movement of the mass fulfils the first law of thermodynamics,
which in this case can be interpreted as:
-
If a constant mass has been moved on a closed path in a gravity field, the
energy is zero.
By this, the mass and its closed path cannot be the reason of the expected
overunity behaviour of this PMM. As all these concepts claim that this behaviour
is caused by the buoyancy effect, we will look at it now.
The Up and Down Movements
First, we analyze the steps 1 and 3 of the circular path. We should remember
how buoyancy works: The forces acting on an immersed mass are generated by
the differences of pressure on top and bottom of this mass. If a mass sinks
or raises, the equivalent volume of liquid has to be forced to the place
in which originally the mass was located.
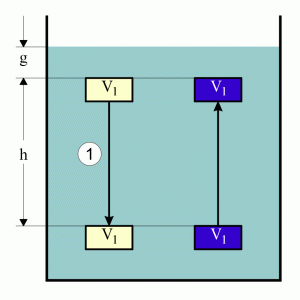 |
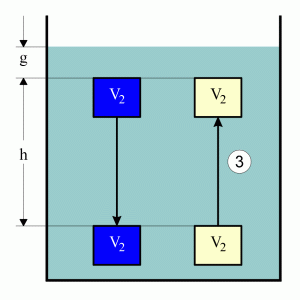 |
| Step 1: The mass sinks. By this, the equivalent volume V1
of liquid moves upwards. |
Step3: The mass raises. So the equivalent volume V2 of liquid
moves down. |
We can conclude, that by this the volume difference
V2-V1 moved down, whereas the mass itself returned
to the original height it had at the beginning of step 1. Obviously, by raising
of the body, a certain mass of liquid moved down without investing energy.
We have found the source of perpetual motion!
Did we?
The Volume Changes
Our analysis has ignored the steps 2 and 4, a point which is frequently
overlooked by inventors of these machines. Let's start the analysis in the
same way as we did with steps 1 and 3 before:
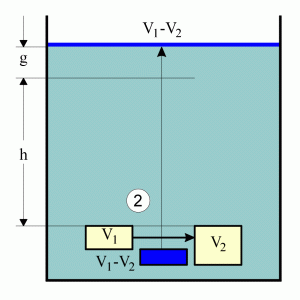 |
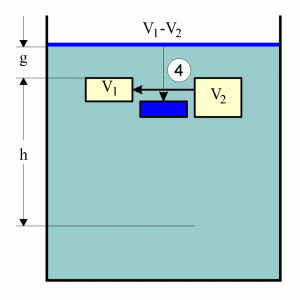 |
| Step 2: The liquid equivalent of volumes V2-V1
moves up |
Step 4: The liquid eqivalent of volumes V2-V1 moves
down. |
Our quick-minded inventor argues, that moving the constant volume up
and down will compensate, thus still leaving the result from or previous
analysis untouched. But slow down! We must be more careful!
We have ignored the work, that is needed to move the liquid. Let's regard
the liquid moving down in step 3 consists of the portions
V2-V1 and V1. It is easy to see, that the
V1 part exactly compensates the movement of the V1
part in step 1. Let's focus on the V2-V1 portion. We
see:
-
in step 2, the volume V2-V1 is moved by the height
h + g, which needs a certain amount of work
-
in step 3, the volume V2-V1 of liquid moves down by
h, thus providing some work.
-
in step 4, the volume V2-V1 of liquid moves down by
g, thus again providing some work
Now we have pushed the liquid mass equivalent to
V2-V1 up by h+g, then moved it down by h and g. The
resulting energy sum is zero. What is the conclusion of this lenghty discussion?
We have proved that the easily ignored steps 2 and 4 i.e. changing of the
volumes are the key to understanding the flaw in this machine. Sorry. No
perpetual motion machine!
The Simplest Explanation
Remember the first law of thermodynamics? Remember the sentence a few lines
above:
-
If a constant mass has been moved on a closed path in a gravity field, the
energy is zero.
Which masses are involved in this circular process?
-
The mass of the immersed body. We've already found out that this particular
mass on its circular path has an energy bilance of zero.
-
The mass of the displaced liquid. When the immersed body retuns to the same
position at the same volume (whichever) on its closed path, the same volume
eqivalent (=mass equivalent) has also moved on a circular path to its original
position. We see, that the energy bilance is zero as well.
We can add zero energy as long and often as we want, the result will never
become positive. Even if the discussed machine is initially set into motion,
it soon will stop, as there are losses by liquid friction, which we have
neglected up to now.
If you are interesed in a more detailled discussion of unworkable buoyancy
machines, have a look at Donald Simanek's
site
on buoyancy misconceptions. If you see any parallelities in ideas, do
not worry! For sure this is no sign for the great conspiration
but rather for scientific collaboration and understanding of basic concepts
in physics.